The Perfect Cadence

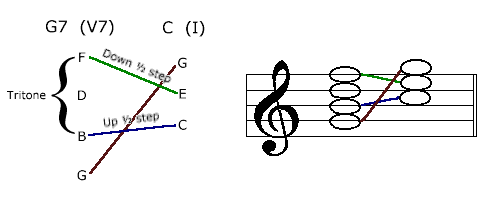
A common chord progression is:	iim7	è	V7	è	I
	subdominant function		dominant function		tonic function

The resultion of the V chord to the I chord is known as the perfect cadence.

The V chord demands resolution because of the tritone interval between the 3 and b7 degrees (tritone = 6 semitones = diminished 5th). This interval is very unstable.

Using G7 to C as an example:

G is the common tone.

The driving forces that impel V7 to I are:

The root of V leaping down a perfect fifth or up a perfect fourth
The third of V rising a semitone (hence its designation leading tone - in G7 to C, B rises to C; 7 to 8 in the C major scale)
The seventh of V, if present, falling a semitone (this greatly increases the thrust of the progression

The Perfect Cadence in a Minor Key

Consider this progression, using natural minor scale harmony:

iiø7	è	vm7	è	im
subdominant function		dominant function		tonic function

The perfect cadence in this progression lacks thrust and so does not demand resolution so readily. If, however, harmony pertaining to the harmonic mionor is used, the following would result:

iiø7

im(

Whilst in this instance the V7 chord demands resolution, the im(7 chord to which it resolves is not a sufficiently stable chord to end on. It is more common practse, therefore, to simply substitute the V7 chord (from the harmonic minor for the vm7 chord (from the natural minor). This gives the following modal choices:

iiø7	è	V7	è	im7
ii Locrian		V Phrygian Major		i Aeolian

Note the extensions available for the V7 chord in a minor key:

V Phrygian Major:		1 b2 3 4 5 b6 b7
V		1, 3, 5
V7		1, 3, 5, b7
V7(b9)		1, 3, 5, b7, b9
V11(b9)		1, 3, 5, b7, b9, b11
V11(b9,b13)		1, 3, 5, b7, b9, b11, b13
V7 sus2		1, 2, 5, 7
V7 sus4		1, 4, 5, 7
		etc.

In a minor key, all these chords could be approached using V Phrygian Major.

Rate This Tutorial

How useful did you find this tutorial?